Concepts addressed: percentages, unit conversion, cylinder volume, cylinder area, equilateral triangle area, regular hexagon area.
Recommended grade: 7th.
Difficulty level: Basic.
A basic version of Building a swimming pool is also available.
Scenario:
This is the year – you’re building an in-ground swimming pool in your backyard! You can already see all the envious faces, but first, someone’s got to do the dirty work.
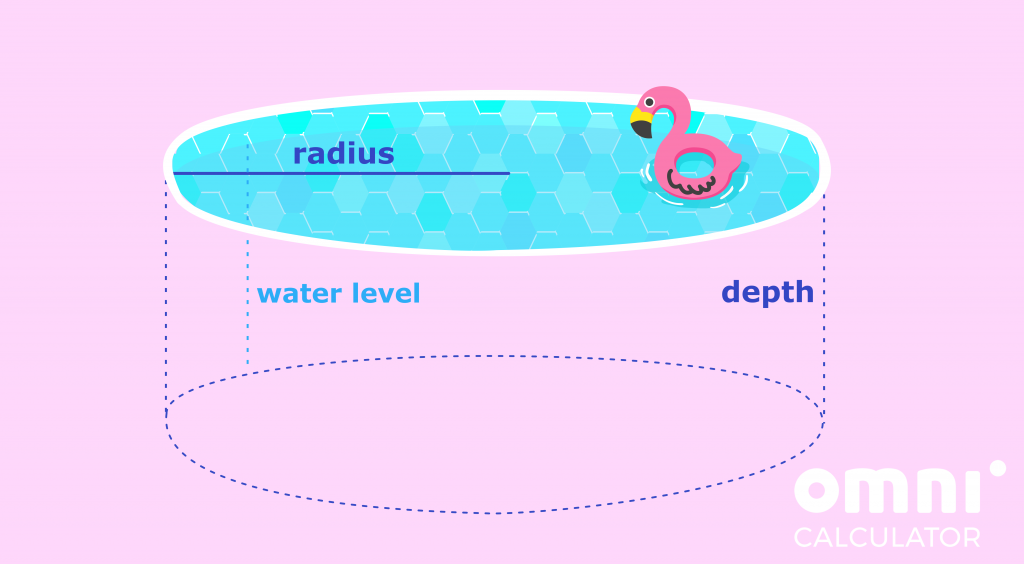
- You’ve decided the pool should be circular, with a radius of six feet and as deep as you are tall. How much water will you need if you want to fill it up nine-tenths of the way (so that it doesn’t spill)?
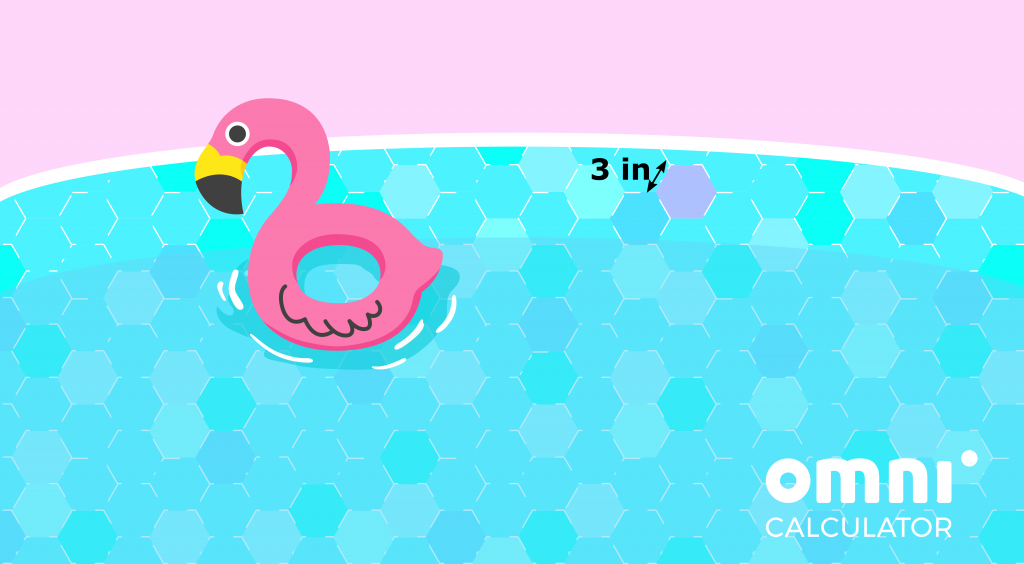
- You want to tile the entirety of the inside with regular hexagonal tiles that have a side 3 in long. How many of those will you need? Better buy 5% more in case some of them break in the process.
- A pack of thirty tiles costs $15, and you get $50 allowance every week. How long will it take to save for them if you already have $80 saved and you’ll be putting all your allowance towards it?
Useful calculators:
- Volume calculator – https://www.omnicalculator.com/math/volume
- Cylinder volume calculator – https://www.omnicalculator.com/math/cylinder-volume
- Circle calculator – https://www.omnicalculator.com/math/circle
- Percentage calculator – https://www.omnicalculator.com/math/percentage
- Hexagon calculator – https://www.omnicalculator.com/math/hexagon
- Conversion calculator – https://www.omnicalculator.com/conversion/conversion-calculator
Question 1 hints:
Question 2 hints:
Question 3 hints:
Solutions (WARNING: depend on the height, example for height being 6 feet ):
Step-by-step solution:
h = h_cylinder * 9/10 = 6 ft * 9/10 = 5.4 ft.
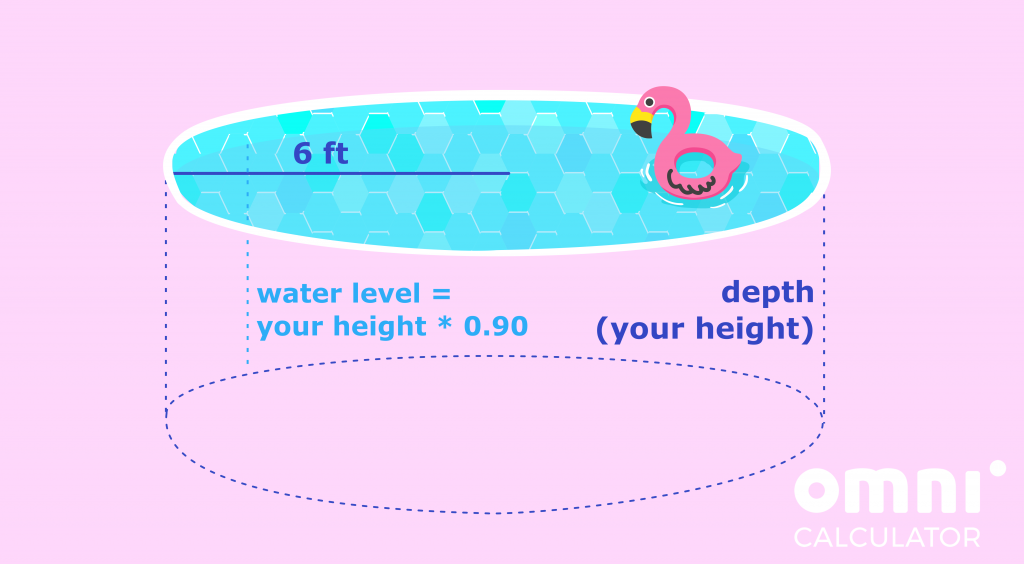
This gives us all the information we need to calculate the volume with the cylinder volume calculator:
V = π * r² * h = π * (6 ft)² * 5.4 ft ≈ 610.4 ft³.
b = 2 * π * r = 12π ft.
Now we can use that same calculator and get the area we want to tile:
A = base_area + lateral_area = π * r² + b * h = π * (6 ft)² + 12π ft * 6 ft ≈ 339.12 ft².

Now that we know how much area we’re working with, we need to get the number of tiles required to tile it. A single tile is a regular hexagon with side a = 3 in. For our calculations, we need the units to agree, so let’s use the conversion calculator to turn a into feet: a = 3 in = 0.25 ft.
Recall that a regular hexagon consists of six equilateral triangles, with sides equal to the hexagon’s side. Also, there is a nice formula for the area of an equilateral triangle that needs only the length of its side. In particular, this means that we are able to calculate the area of a single tile with the hexagon calculator:
A_tile = 6 * area_triangle = 6 * (a² * √3) / 4 = 6 * ((0.25 ft)² * √3) / 4 ≈ 0.16 ft².
Therefore, we’ll need:
A / A_tile ≈ 339.12 ft² / 0.16 ft² = 2,119.5
tiles to cover the whole area. Clearly, you can’t buy half of a tile, so the actual number we need is 2,120.
Lastly, we need to add to that number the additional 5% in case some of the tiles break. If we want to buy 5% more than what is needed, then this means that we need 100% + 5% = 105% of what we calculated above. According to the percentage calculator this is 105% * 2,120 = 2,226 tiles.
2,226 / 30 = 74.2.
Therefore, we’ll need 75 packs (since we cannot just buy 0.2 of a pack). This, in turn, boils down to
cost = 75 * pack_cost = 75 * $15 = $1,125.
Now let’s subtract from that the amount you’ve already saved to get how much we still need:
money_needed = cost – money_saved = $1,125 – $80 = $1,045.
Lastly, we must see how many allowances (and therefore weeks) it will take to save such a sum. Since we get $50 every week, we need 1,045 / 50 = 20.9, or rather 21 weeks, to get that much money. But summer will be over in 21 weeks!
Oh well, maybe next year will be the year?