This scenario tests basic arithmetic and data analysis.
Recommended grade: 6th.
Difficulty level: Basic.
This scenario was originally created by Karolina Semrau, Julia Zyzak, and Martyna Zyzak, students at the Cracow University of Economics. Martyna Zyzak is also a Clinical Dietitian with experience in pediatric nutrition.
We encourage teachers to use this scenario as a way start a discussion about nutrition and the importance of building healthy habits in the classroom!
Scenario:
Suzie is your typical American 18-year-old. Going to school, gossiping with her friends, chilling in the afternoons.
Recently, her school got a new vending machine that quickly became popular with the students. Suzie herself became fond of the candy bar “Omni Munch” and a soda “Calc-Pop” and buys them every day (including weekends – they’re that good). This means additional fats and carbs, but it’s just a snack and a drink, so it can’t be much, can it? Still, whatever we do regularly, affects our health.

- Suzie is 18, she weighs 110 pounds and is 5’3”. So far, she’s eaten healthy and regularly, and took part in P.E. 2-3 times a week.
What is Suzie’s required daily calorie intake?
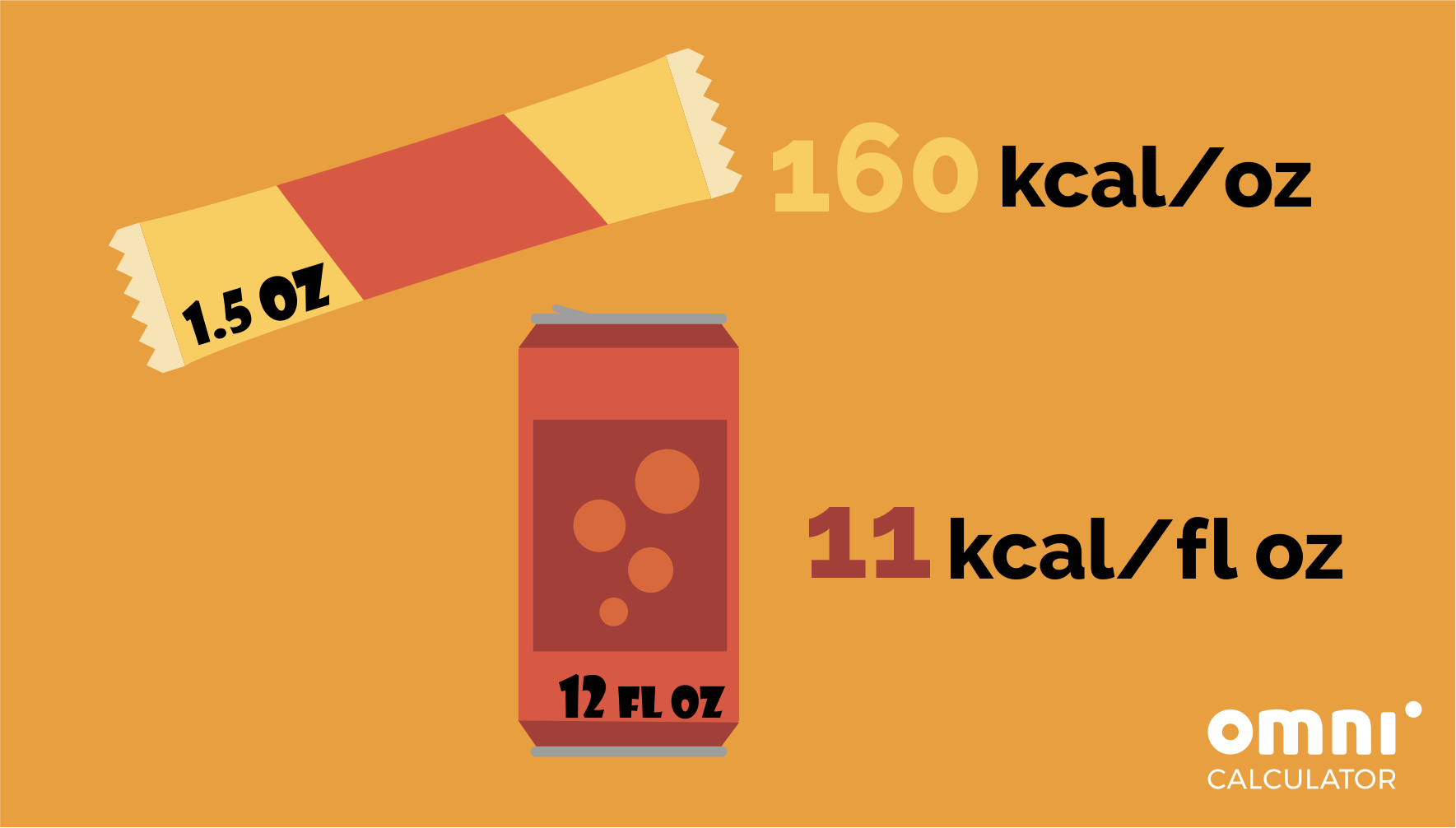
- The candy bar is 1.5 oz and has an energy value of 160 kcal per ounce. On the other hand, the soda can is 12 fl oz, and its energy value is 11 kcal per fluid ounce. If she continues with the new snacks, how many extra calories do they translate to in the course of:
a) a day;
b) a week;
c) a month (30 days);
d) a year (365 days)?
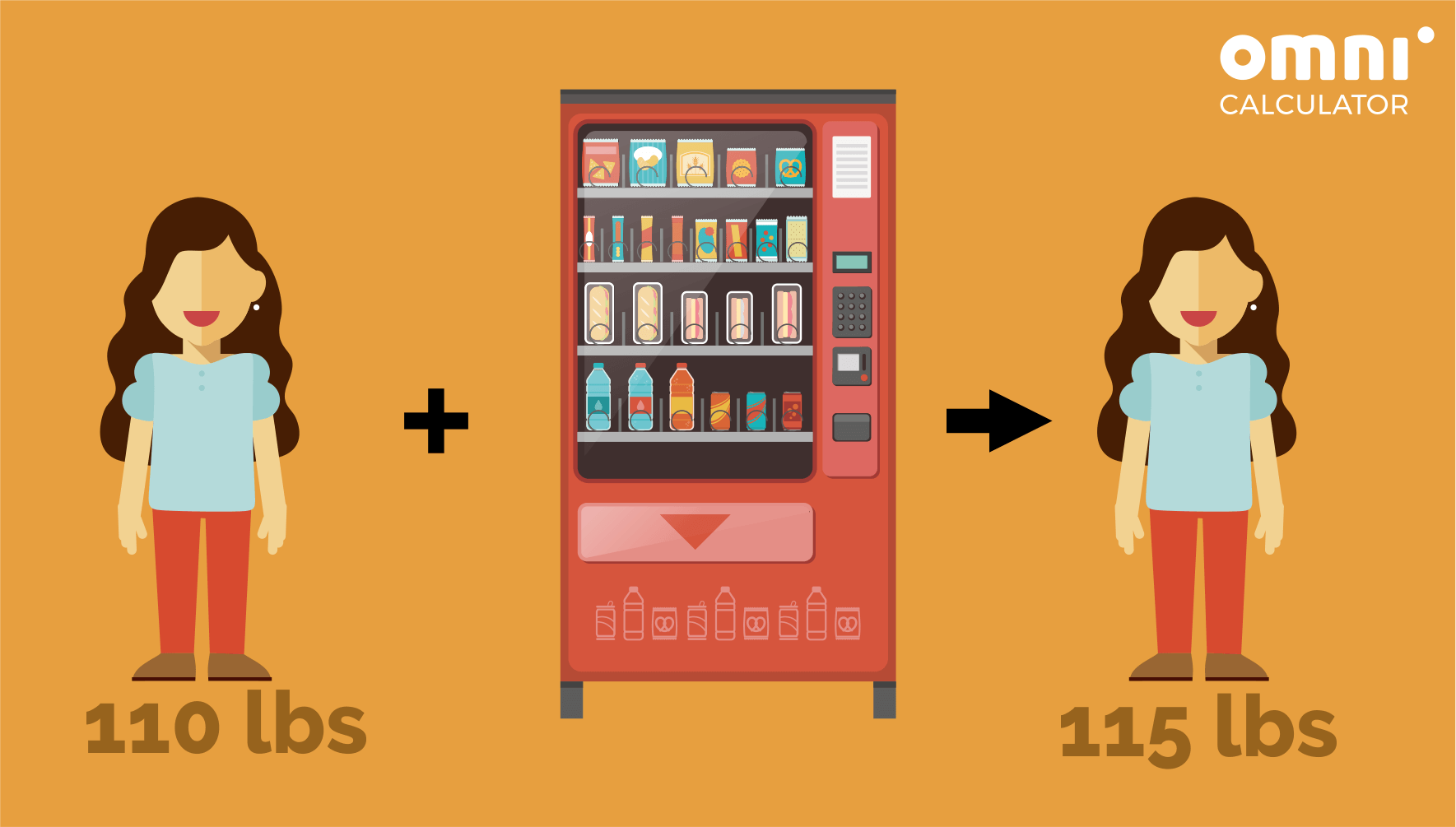
- What is Suzie’s BMI (Body Mass Index) and BMI Prime? Suppose that the vending machine made her put on 5 extra pounds. How does that affect the results?
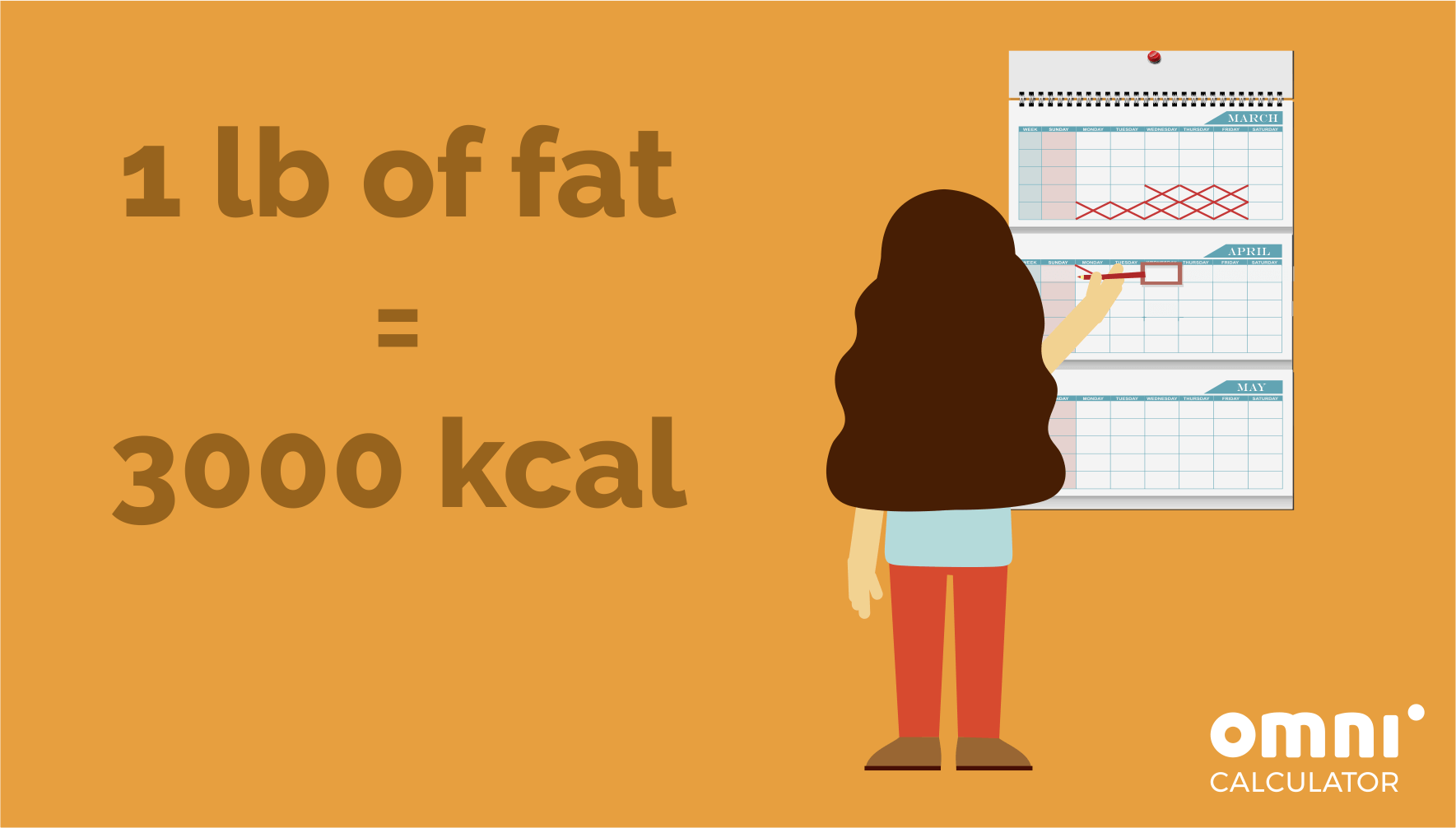
- Before they installed the vending machine, Suzie consumed the number of calories corresponding to her daily needs. Now that she’s added the snacks, she has a calory excess that will turn into body fat.
If one pound of fat is 3,000 kcal, calculate how many days it took Suzie to gain 5 pounds.
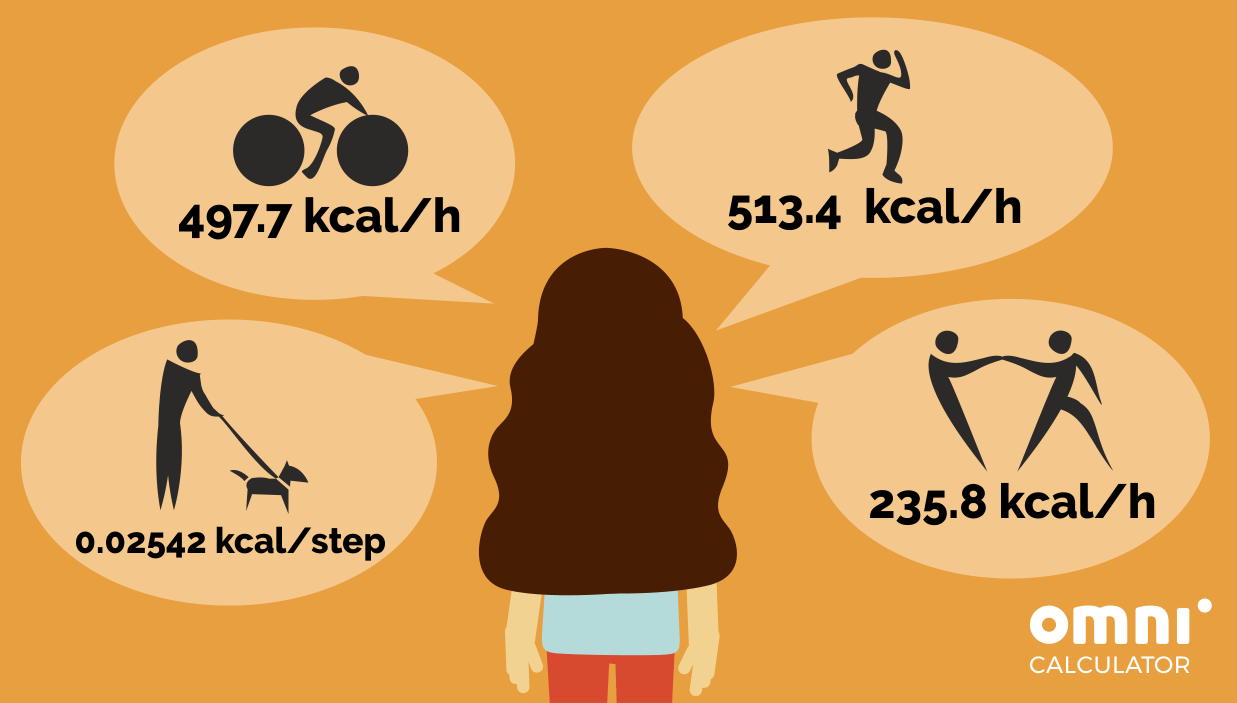
- Suzie decided to do something about the problem and think about extra physical activities to burn the additional calories. How many steps would it take Suzie to do that? Alternatively, how much time would she have to spend:
a) riding a bike;
b) jogging;
c) dancing?
Useful calculators:
- Conversion calculator – https://www.omnicalculator.com/conversion/conversion-calculator
- Calorie calculator – https://www.omnicalculator.com/health/calorie
- BMR calculator – https://www.omnicalculator.com/health/bmr
- BMI calculator for teens – https://www.omnicalculator.com/health/bmi-teens
- Steps to calories calculator – https://www.omnicalculator.com/sports/steps-to-calories
- Calories burned calculator – https://www.omnicalculator.com/sports/calories-burned
Question 1 hints:
Question 2 hints:
Question 3 hints:
Question 4 hints:
Question 5 hints:
Solutions:
b) 2,604 kcal;
c) 11,160 kcal;
d) 135,780 kcal?
After gaining: BMI = 20.4, BMI Prime = 0.82.
The numbers increased, but Suzie’s still considered normal/healthy. Still, if she continues like this, she might approach being overweight.
a) 44 mins;
b) 43 mins;
c) 1h 34 mins.
Step-by-step solution:
BMR(women) = (10 * weight / 1kg + 6.25 * height / 1cm – 5 * age / 1 year – 161) kcal / day.
Observe that we’ll need her weight, height, and age. In our case, they are weight = 110 lbs, height = 5 ft 3 in, and age = 18 years. However, the formula uses kilograms and centimeters, which means that we need to change the data into those units. Using the conversion calculator, we obtain
weight = 110 lbs ≈ 49.9 kg,
height = 5 ft 3 in ≈ 160 cm.
Then, the BMR calculator gives that
BMR(Suzie) ≈ (10 * 49.9kg / 1kg + 6.25 * 160cm / 1cm – 5 * 18 years / 1 year – 161) kcal / day = 1,248 kcal/day.
Lastly, we need to take into account Suzie’s lifestyle, i.e., that she does physical activity 2-3 times a week. This means that we need to multiply the BMR above by a factor of 1.6:
recommended_intake = BMR(Suzie) * 1.6 = 1,248 kcal/day * 1.6 ≈ 1,997 kcal/day.
WARNING: We could have also used the calorie calculator straight away by simply changing the units there. This would make the calculator change the units by itself, sparing us some work. However, the answer it then gives is 2,012 kcal/day. The discrepancy between the two results is due to rounding up the numbers when changing feet to centimeters and pounds to kilograms.
candy_bar = 1.5 oz * 160 kcal/oz = 240 kcal,
soda = 12 fl oz * 11 kcal/fl oz = 132 kcal.
This means that their combined calorie content is
daily_calories = candy_bar + soda = 240 kcal + 132 kcal = 372 kcal.
The above number is how many calories from the vending machine Suzie consumes daily. If we now want to see how many it will be in a week, a month, and a year, we need to multiply the above value by the number of days that correspond to our question: 7, 30, and 365, respectively. We get
weekly_calories = 7 * 372 kcal = 2,604 kcal,
monthly_calories = 30 * 372 kcal = 11,160 kcal,
yearly_calories = 365 * 372 kcal = 135,780 kcal.
BMI = weight / height²,
BMI_Prime = BMI / 25.
However, we need to be careful. The above formula for BMI uses kilograms and meters instead of pounds and feet. Therefore, we need to begin by changing the units with the conversion calculator:
weight = 110 lbs ≈ 49.9 kg,
height = 5 ft 3 in ≈ 1.6 m.
Also, we’ll need the weight of Suzie after gaining the few pounds:
weight_after = 115 lbs ≈ 52 kg.
Now we’re ready to do the calculations. We start with the ones before the weight gain:
BMI_before = 49.9 kg / (1.6 m)² ≈ 19.5,
BMI_Prime_before = 19.5 / 25 = 0.78.
And now the ones after:
BMI_after = 52 kg / (1.6 m)² ≈ 20.3,
BMI_Prime_after = 20.3 / 25 = 0.81.
Both of the above results fall into the “normal/healthy” category, so Suzie should be fine for now. However, we can imagine that continuing her unhealthy snack habit may lead to her being overweight.
WARNING: Just as in Question 1, we could have simply changed the units in the BMI calculator for teens and make it do all the unit conversions for us. It would then spit out the answers as:
Before gaining: BMI = 19.5, BMI Prime = 0.78
After gaining: BMI = 20.4, BMI Prime = 0.82.
Again, the discrepancies are a result of rounding up the numbers when changing units.
5 * 3,000 kcal = 15,000 kcal.
Recall from Question 2 that the candy bar and the soda together contain 372 kcal. Now, if we want to know how many days it took Suzie to gain the 5 pounds (i.e., consume the extra 15,000 kcal), all we need to do is divide the two numbers:
15,000 kcal / 372 kcal ≈ 40.3,
which means that it took Suzie 41 days.
We’d like the girl to burn the 372 kcal that come from the candy bar and the soda. Therefore, to calculate how many steps she’d need to take daily, it’s enough to divide the two numbers:
steps = 372 kcal / 0.02542 kcal ≈ 14,634.
Next, we move on to other physical activities. With the calories burned calculator we can see how many calories Suzie burns during one hour of cycling, running, and dancing. Note, that she did gain 5 pounds, so her weight is 110 lbs + 5 lbs = 115 lbs. This gives us the following numbers:
cycling = 497.7 kcal/hr,
running = 513.4 kcal/hr,
dancing = 235.8 kcal/hr.
We want how much activity is needed to burn the 372 kcal from the snacks. Therefore, we need to divide that number by each of the ones from the list above:
time_cycling = 372 kcal / 497.7 kcal/hr ≈ 0.75 hr ≈ 45 min,
time_running = 372 kcal / 513.4 kcal/hr ≈ 0.72 hr ≈ 43 min,
time_dancing = 372 kcal / 235.8 kcal/hr ≈ 1.58 hr ≈ 95 min.
Warning: If we decide to skip researching how many calories Suzie burns with every step and with every hour of some activity, and simply input the data into the steps to calories calculator and the calories burned calculator, but be aware they may spit out slightly different answers. For example, the answer to the first question would then be 14,633 steps. Just as before, this difference is due to rounding up the numbers in between. It’s one small step for Suzie, but one giant leap for keeping fit.